CHAPTER FIVE
Competence Without Comprehension
Not even three months into my quantum experiment, my oscillations between giddiness and gloom are trending downward. To shore up my wobbling resolve, I post a column on Scientific American about my effort to “grasp quantum mechanics the way physicists do.” It will be harder to abandon this project now that I’ve announced it. I’m getting feedback, ranging from pats on the back to slaps across the face. A physicist’s comment on Facebook is typical: “The naiveté is almost insulting, having so little understanding of the amount of work it takes to master the mathematics and theory required.” Fuck ‘em, I think, but the criticism rattles me.
Alex Wellerstein, an historian of science at my school, says on Facebook: “Are you brave, foolish, or both at the same time, depending on how you measure it?” A cute allusion to Schrodinger’s dead/alive cat, and a reasonable question. Perhaps I can convince myself and others that I’m brave as long as I don’t get tested. Quantum mechanics implies that if you don’t watch something happening, it’s not really happening. Donald Trump adheres to this logic. He says we shouldn’t get tested for the Covid-19 virus, because testing makes our cases go up. Trump’s comment, Sabine Hossenfelder says on her blog, “demonstrates his deep knowledge of quantum mechanics.”
Hossenfelder also comments on my quantum project. “Soon enough,” she says on Facebook, Horgan “will have his own personal interpretation named after him.” She’s teasing me, but I find her comment inspiring. When science-y people insult me, I like to embrace the insult. Philip Anderson, a Nobel laureate in physics, loathed The End of Science, which he thought might become a self-fulfilling prophesy by discouraging young, would-be scientists. He coined the term Horganism to describe unwarranted pessimism about science’s future. I made Horganism my Twitter handle. [1]
Maybe someday I’ll invent my own interpretation of quantum mechanics, even though it’s likely to be met with ridicule or, more likely, indifference. First, I need to learn the damn theory. I’m dipping once again into Leonard Susskind’s Quantum Mechanics: The Theoretical Minimum: I hope it will make more sense now that I’ve brushed up on calculus and linear algebra. I review Susskind’s discussion of how quantum mechanics makes use of complex numbers, which combine real and imaginary numbers. Here’s my stab at a synopsis. Warning! Jargon ahead!
Each complex number has a corresponding complex conjugate, which reverses the sign of the imaginary component. Hence the complex conjugate of z, if z = x + iy, is x – iy. Complex numbers and their conjugates serve as the basis of a system invented by Paul Dirac for representing position, momentum, spin and other quantum properties. Dirac’s system caught on because it simplifies quantum calculations—a claim I must take on faith.
Dirac’s system employs bracket notation, so-called because the names of vectors are enclosed in brackets, namely | > or < |. Dirac calls the basic components of his system “bras” and “kets.” “Bra” plus “ket” equals bracket, get it? Let’s start with the ket. It consists of a list of complex numbers, usually represented as a vertical column, in Hilbert space, where real and imaginary numbers roam.
Together, the complex numbers specify a vector, written as |V> in Dirac notation, that tracks some behavior or property of, say, an electron. |V> corresponds, in an abstract, indirect way, to the superposed probabilities of what you will see when you look at the electron: It will veer right or left or have a spin of up or down. |V> is the ket.
Now take the ket’s vertical column of numbers, lay it on its side and replace the complex numbers with their complex conjugates. That produces a new vector, the bra, indicated with reverse bracket notation: <V|. You then multiply the bra times the ket using the rules of matrix multiplication. This operation, which is called taking the inner product of the vector, gives you a new vector, which brings you closer to computing the probability that an electron will go left or right or whatever.
All this is confusing enough. I really hit the wall when I get to Hermitian operators. Jim Holt, my quantum advisor, says Hermitian operators are the key to quantum mechanics. Yes, another key, along with differential equations, matrices and bras and kets. Physicists are like janitors, walking around with giant key rings jangling on their hips.
A Hermitian operator is a special kind of matrix. Let’s say the Hermitian operator is a 3 x 3 matrix, consisting of three rows and three columns of numbers, nine numbers in all, which can be complex or real. Draw a diagonal line from the top left number to the bottom right number. If you flip the matrix around this diagonal axis, and you simultaneously complex-conjugate everything, you get the same matrix.
This symmetry of Hermitian operators is crucial for connecting the abstractions of complex numbers in Hilbert space to physical events in our three-dimensional world. Leonard Susskind compares a Hermitian operator to a “machine” with an input port and an output port. You feed vectors into the machine, and the machine pops out new vectors. Hermitian operators, plus other mathematical maneuvers, do certain things for you. First, they ensure that all the probable, superposed behaviors of the electron add up to a probability of one; this property is known as unit normalization.
Second, they ensure that vectors representing logically exclusive values of specific properties, such as position, are orthogonal, which is roughly equivalent to perpendicular. Orthogonality underpins mutual exclusivity; if you pinpoint the electron’s position, then the probability of it being anywhere else vanishes.
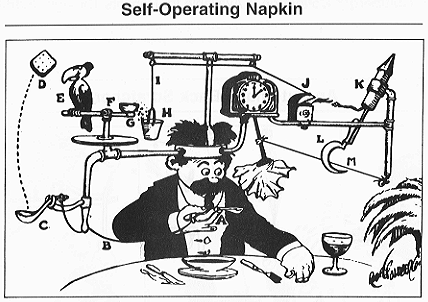
This is an artist’s conception of a Hermitian operator. No, wait, it’s Rube Goldberg’s automatic mouth-wiping device. Source: Wikipedia.
Third, Hermitian operators help turn complex numbers into real numbers corresponding to positive probabilities. Hermitian operators get rid of the imaginary components of vectors through something called the spectral theorem, which defies my comprehension. In all these ways, the Hermitian operator helps link the imaginary quantum realm to the real world, where physicists with huge key rings lumber around in labs. [2]
I can write down these peculiar facts, but they do not add up to anything resembling understanding. Like Feynman’s whirligigs, which help you calculate the odds that a photon will bounce off glass, Hermitian operators mystify me. They’re black boxes with magical powers, like my TV or smartphone, except even more opaque. Physicists gush over the beauty and elegance of their mathematical models, but quantum mechanics seems increasingly awkward and jury-rigged. The theory resembles one of those absurdly complicated contraptions invented by Rube Goldberg for accomplishing simple tasks, like wiping your face with a napkin. But the damn thing works.
The Math Instinct
Early on in this project, I recalled with incredulity the hypothesis that we have an innate talent for math bred into us by natural selection. This “number sense” or “math instinct” is supposedly analogous to our innate capacity for language. Slogging through Quick Calculus I thought, Math instinct? Give me a break. Only a tiny, freakish minority can perform chain-rule calculations effortlessly, intuitively, the way the rest of us exchange insults with lovers.
Math at work: clock tower at Hoboken terminal keeps time even on a misty day.
But lately, I’ve become acutely conscious of how deeply numbers are embedded in our everyday lives. Take my morning exercise routine. While jogging, I keep track of my circuits around Hoboken Park (which isn’t hard, because I usually stop after two). I glance at the Hoboken clock tower: 8:35, better get home soon to make my 10 a.m. dentist appointment. I know that today is Tuesday, second day of the week, and that tomorrow afternoon, 4-ish, I’ll head over to Manhattan to see Emily.
I check NYTimes.com every morning for trends in Covid cases and deaths. Are things getting better or worse? Emily recently made me buy an oximeter to make sure the coronavirus hasn’t invaded my lungs. This morning, the red digital readout says my oxygen is between 95 and 97 percent, which means I’m not dying. At least once a day I visit a website that tells me, with graphs and percentages, whether my retirement account is going up or down.
Also, many routine chores—brushing teeth, washing dishes, walking up or down stairs—are enormously complex feats requiring countless calculations. Robots would be hard-pressed to replicate these tasks. And yet our brains carry out the computations rapidly, automatically, without dragging them into our awareness for our appraisal and approval. Maybe I do have a math instinct, and maybe it’s based on some neural equivalent of a Hermitian operator. Unfortunately, that instinct can’t help me understand Hermitian operators.
The Chinese Room
I began this experiment hoping to understand quantum mechanics, or at least to understand why I can’t understand. I saw this project as a route to deeper insights into physics and nature. But something odd has been happening to me. I’m beginning to wonder whether anyone really understands anything. The Chinese room experiment keeps coming to mind.
Philosopher John Searle proposed this thought experiment back in the 1980s to convince us that computers don’t consciously “think” as we do; they just manipulate symbols mindlessly without knowing what they are doing. But to my mind, his thought experiment implies that we humans can be pretty mindless too, even when engaged in a pursuit as lofty as quantum physics.
Searle asks us to imagine a man who doesn’t understand Chinese sitting in a room. The room contains a gigantic manual of rules, which specify how to respond to a string of Chinese characters with another string of characters. Someone outside the room slips a sheet of paper with Chinese characters on it under the door. The man finds the appropriate response to the cryptic message in the manual, copies it onto a sheet of paper and slips it back under the door.
Unknown to the man, he is replying to a question like “What’s your favorite color?” with an appropriate answer: “Blue.” In this way, he mimics someone who understands Chinese even though he doesn’t. That’s what computers do, according to Searle. They process symbols in ways that simulate human thinking, but they are just automatons mindlessly following rules. Searle’s thought experiment has provoked countless objections. Here’s mine: The Chinese room experiment is a splendid case of begging the question--not in the sense of raising a question, which is what most people mean by the phrase nowadays, but in the original sense of circular reasoning.
The meta-question posed by the Chinese room experiment is this: How do we know whether any entity, biological or non-biological, has a subjective, conscious experience? When you ask this question, you are bumping into what I call the solipsism problem. As Thomas Nagel pointed out in “What Is It Like to Be a Bat?”, no conscious being has direct access to the conscious experience of any other being. I know I’m conscious, but I can’t be absolutely sure that you or any other person is conscious, let alone a jellyfish or bat or smartphone. I can only make inferences based on the behavior of the person, bat, smartphone or whatever.
I assume that most humans, including readers of this book, are conscious, as I am, but I can’t know for sure, because of the solipsism problem. Nor can I know what it’s like to be the man in the Chinese Room. He may or may not understand Chinese. He may or may not be conscious. There is no way of knowing, again, because of the solipsism problem. Searle assumes that we can know what is going on, or not going on, in the man’s mind, and hence, by implication, what’s going on or not in a machine. His flawed initial assumption leads to his flawed, question-begging conclusion that machines can’t think as we do.
What does the Chinese room experiment have to do with my quantum experiment? Here’s what. Physicists insist that you can’t understand quantum mechanics without understanding its underlying mathematics. But according to Richard Feynman, even if you master the math, you still won’t understand quantum mechanics! Not only that, but physicists who focus relentlessly on the math, who shut up and calculate, might end up like the guy in the Chinese room, mindlessly processing symbols without knowing what they mean.
This possibility reminds me of the argument of philosopher Daniel Dennett that consciousness is overrated, at least when it comes to doing what we need to do to get through a typical day. We carry out tasks with little or no conscious attention, like virtual zombies. Dennett calls this zombie-like behavior “competence without comprehension.”
I’ve criticized Dennett’s claim, vehemently. Dennett might be a zombie, I harrumph, but I’m not! And so on. But the more I think about Dennett’s argument, the more sense it makes. Our minds are habituation machines, designed to turn even complex tasks—from manipulating a Hermitian operator to appeasing a lover--into routines that we perform by rote, with minimal cognitive effort.
The Chinese room serves as a metaphor not only for physics but also for the human condition. Each of us sits alone within the cell of our subjective awareness. We keep receiving cryptic messages from the outside world. Only dimly comprehending what we are doing, we compose responses and slip them back under the door. In this way, we manage to survive, to get through the day, even though we never really know what is happening. [3]
QBism
I’m only momentarily heartened when I get an email from Chris Fuchs, a physicist whose name I recognize from online quantum chitchat. Fuchs saw my column on my quantum experiment, and he wants to give me encouragement. He’s been trying to understand quantum mechanics since he first encountered it in college. “Good luck in your experiment!” he writes. “You may never emerge, as I haven’t!”
Fuchs has written a book about his own experiment, Coming of Age with Quantum Information, that includes extended email exchanges between him and other physicists. The book reveals the origins and evolution of QBism, an interpretation of quantum mechanics that Fuchs helped invent. QBism is pronounced “Cubism,” and like the art movement it offers a cracked-mirror view of reality.
This drawing evokes John Wheeler’s suggestion that our observation of the universe brings it into existence, kind of.
Fuchs says QBism was inspired in part by the quantum conjectures of John Wheeler. Musing over the measurement problem, Wheeler speculated that we live in a “participatory universe” shaped by our observations of it. In a chapter of Coming of Age titled “Participatory Realism,” Fuchs notes that physics, historically, has sought to explicate “the impersonal laws of nature.” But since the advent of quantum theory, there has been “a nagging pressure to insert a first-person perspective into the heart of physics.”
QBism yields to that nagging pressure. Rather than a theory of reality, QBism is a theory of our beliefs about reality. It is sometimes said to stand for Quantum Bayesianism. Bayesianism is a mathematical method for updating beliefs based on new evidence. That, at any rate, is my tentative understanding of QBism. Reading Fuchs, I have a hard time grasping the theory, in part because it is a moving target; it keeps evolving as Fuchs and others keep talking about it. QBism isn’t a theory, it’s a conversation.
David Mermin, coiner of Shut up and calculate, is a fan of QBism. He defends it in an essay, “Making Better Sense of Quantum Mechanics.” Mermin points out that all our knowledge begins with subjective, conscious experience. Each of us constructs a picture of the world, a set of beliefs about it, based on our interactions with it. We constantly, implicitly, assign probabilities to our beliefs, which we adjust in response to others’ beliefs.
QBism gets rid of the measurement problem and other quantum paradoxes; you just need to accept the theory’s radical subjectivity. QBism makes individual subjective experience the bedrock of reality. QBists hedge their bets, if only so they don’t come across as loons or mystics. QBism doesn’t deny the existence of matter; it says that objective, material reality emerges from our individual perceptions of it.
Given their embrace of subjectivity, Fuchs and other QBists should accept that many people will, for subjective reasons, reject QBism. Part of me, the hard-headed, sensible part, is leery of QBism and other interpretations that make mind fundamental. They strike me as throwbacks to the pre-scientific era, when we simply assumed because of our innate narcissism that the universe revolves around us. Theories that emphasize subjectivity can also be dangerous, especially when many people—like, say, the President of the United States of America--disdain the scientific consensus on climate change and vaccines and even the whole idea of objective “facts.”
The Cult of Bayes
Back for a moment to Bayesianism, the “B” of QBism (although Chris Fuchs now says that QBism just stands for itself, like KFC, formerly known as Kentucky Fried Chicken). Many scientists, including physicists, have embraced Bayesianism with cultish fervor, claiming that it can help distinguish truth from falsehood. Wouldn’t that be great if it were so? Artificial-intelligence researchers employ Bayesian algorithms to help machines recognize patterns and make decisions, such as distinguishing cats from dogs or valid emails from spam. Cognitive scientists conjecture that our brains incorporate Bayesian calculations.
I looked into Bayesianism a while back to see what the fuss was all about. Here’s the story. In the 18th-century, the scholar-cleric Thomas Bayes invented a theorem, now named after him, for calculating the validity of beliefs based on available evidence. The formula takes this form:
P(B|E) = P(B) X P(E|B) / P(E)
Sheldon riffs on Bayes theorem on the nerdy sitcom Big Bang Theory.
P stands for probability, B for belief and E for evidence. P(B) is the probability that B is true, and P(E) is the probability that E is true. P(B|E) means the probability of B if E is true, and P(E|B) is the probability of E if B is true.
English translation: The probability that a belief is true given new evidence equals the probability that the belief is true regardless of that evidence times the probability that the evidence is true given that the belief is true divided by the probability that the evidence is true regardless of whether the belief is true.
That sounds complicated, but Bayes theorem is just a codification of common sense. It says that the plausibility of your belief depends on the degree to which your belief--and only your belief--explains the evidence for it. The more alternative explanations there are for the evidence, the less plausible your belief is. Embedded in Bayes theorem is a moral message: If you aren’t scrupulous in seeking alternative explanations for your evidence, the evidence will just confirm what you already believe. Bayes theorem says: Doubt yourself.
Ironically, scientists use Bayes theorem to confirm rather than challenge their biases. Believers in string and multiverse theories add a dash of Bayes to make the theories appear more credible. A Bayesian statistician at Harvard has helped tobacco companies fight lawsuits from smokers. Bayes theorem isn’t a magic wand; it is a tool that can serve any cause, good or bad. A formula for telling truth from bullshit? Tell me another one.
The Ghost of Ptolemy
Another gripe. The more I learn about quantum mechanics—about bras and kets and Hermitian operators--the less inevitable the theory seems. It resembles an invention like the steam engine—or Rube Goldberg’s face-wiping device—rather than a discovery like pi. Quantum mechanics did not pre-exist us, waiting in the Platonic ether for us to discover it, any more than the cotton gin did. The theory seems jury-rigged, ad hoc, kludgy. “Kludgy” is a computer programmer’s derogatory label for inefficient, inelegant software.
Susskind points out that a key advance depended on Paul Dirac’s realization that quantum functions have much in common with Poisson brackets. Invented by Simeon Denis Poisson, Poisson brackets are a clever way to track the energy of a system. If that piece of 19th-century math hadn’t been lying around, or if Dirac hadn’t known about it… Yes, quantum mechanics works, fantastically well, but does it really mirror the world?
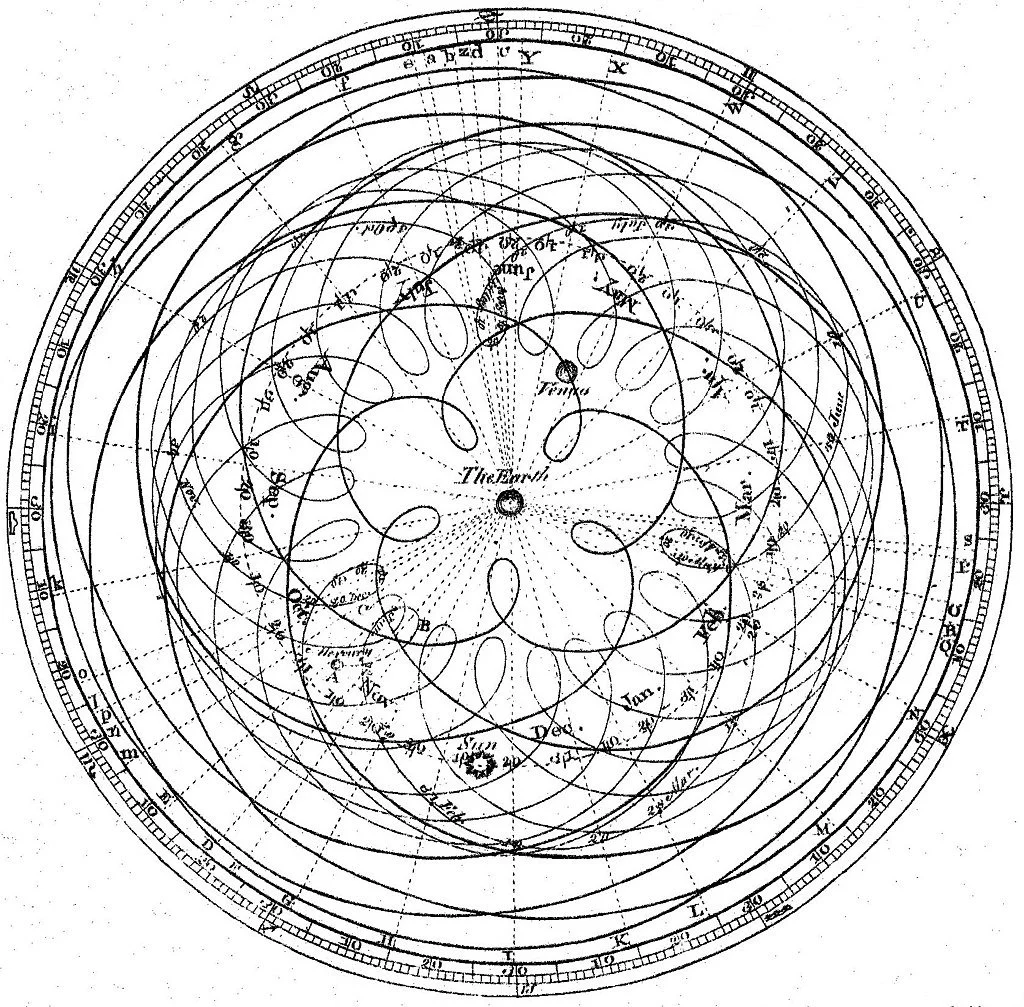
Ptolemy comes to mind. Ptolemy was the genius of geocentrism. His model of the solar system was kludgy, a hodgepodge of ad hoc rules, all based on the assumption that Earth is at the center of things. In Ptolemy’s cosmos, the Sun and planets, not to mention the Moon, whirl around us in improbably baroque cycles and epicycles. We see Ptolemaic astronomy today as the embodiment of scientific wrongness, but it worked; it accurately predicted planetary motions as well as solar and lunar eclipses.
Ptolemaic-style orbits of Venus, Mercury and the Sun. They’re kind of beautiful, actually. From Wikipedia.
Could physics undergo a paradigm shift, equivalent to the switch from geocentrism to heliocentrism, that solves the paradoxes of quantum mechanics? That makes everything much clearer and simpler? Thinking about this possibility, I remember something Stuart Kauffman, a complexity theorist and would-be biological revolutionary, once told me.
The paradigm of evolutionary theory plus genetics, after more than a century of revision, elaboration and application, has accumulated enormous inertia, Kauffman said. Getting biologists to give serious consideration to alternative paradigms is difficult. The neo-Darwinian paradigm works really well, and it has the weight of tradition, custom and indoctrination behind it. So what hope is there, Kauffman asked with grim resignation, for iconoclasts like him?
This argument is even more true of quantum mechanics. Although it lacks the explanatory clarity of natural selection, quantum theory works. It has spawned industries. The more time passes, the less likely it seems that a bright, ambitious young scientist will have the inclination, let alone the intellectual power and force of personality, to upend the quantum paradigm.
That paradigm, although it makes no sense, is brutally, suffocatingly dominant. The wildly diverse interpretations of quantum mechanics, far from threatening its dominion, reinforce it. The great John Bell once said that quantum mechanics “carries in itself the seeds of its own destruction.” Marx said the same thing about capitalism, and yet here we are. Like capitalism, quantum mechanics devours its dissidents.
Wigner’s Friend
A kerfuffle has broken out on Facebook over the “Wigner’s friend paradox.” The paradox refers to a thought experiment proposed in 1961 by Eugene Wigner. His experiment is a variation on Schrodinger’s cat. Instead of a cat in a box, imagine a person in a laboratory, Wigner’s friend, who is monitoring a radioactive specimen. When the specimen decays, a detector emits a flash of light.
Then imagine that Wigner is standing outside the lab. If Wigner’s friend looks at the detector in the lab, he collapses the wave function of the particle and observes whether the particle has decayed or not. But to Wigner, outside the lab, his friend and the entire lab remain in a state of superposition. There seem to be two realities: the reality of Wigner’s friend, and the reality of Wigner.
Physicists have reportedly performed a version of Wigner’s experiment and shown that his intuitions were correct. George Musser says in Science that the experiment calls “objectivity” into question. Musser writes, “It could mean there is no such thing as an absolute fact, one that is as true for me as it is for you.” Science is a leading journal, and Musser is an excellent physics writer, a real pro, with a graduate degree in planetary physics.
Experts, including a physicist involved in the recent Wigner’s-friend experiment, are bickering about its meaning on Facebook. The squabbling seems to confirm Musser’s takeaway: There is no objectivity, there are only subjective, first-person viewpoints. Just as Chris Fuchs, David Mermin and other QBists have been saying.
So what’s the point of arguing about what quantum mechanics means? To argue is to assume, implicitly, that you might reach some sort of objectively valid resolution. If you’ve already established that the argument can’t be resolved in this way, why argue? What’s the point? It’s like anarchists arguing over how to govern. The whole exercise is oddly self-contradicting.
Also, the debate seems silly. Yes, scientists can be biased, but they are still capable of discovering objective truths. Scientific objectivity has been demonstrated over and over again in innumerable ways, as evidenced by hydrogen bombs, jets, smartphones, antibiotics and other advances. Quantum mechanics can at best force philosophers, when they are talking about objectivity, to add a few caveats to their comments. But the end of objectivity? Come on, get real.
Emily, after listening to me babble about Wigner’s friend and the end of objectivity, asks if I ever read the commencement speech that novelist David Foster Wallace gave at Kenyon College. Yeah, probably, I reply. I’m annoyed by her digression, hoping she’ll drop it. But my girlfriend doesn’t drop it; she never drops it. She reads Wallace’s speech to me, and it blows me away. Here’s my paraphrase:
All of us are trapped in the prison of ourselves. We have a tendency to see other people as obstacles to our happiness and self-fulfillment, when in fact, like us, they’re just trying to squeeze a little pleasure out of life. You don’t have to see life and other people in such a negative way. Let’s say you’re in a supermarket, alone, buying your dinner. Instead of loathing the other shoppers and seeing them as brain-dead zombies, you might use your imagination to feel compassion and empathy for them, even a mystical connection. That might be an illusory feeling, but maybe it’s real, and it helps you escape from the horror of your frustrating, soul-crushing existence.
This speech is made more poignant by our knowledge that Wallace’s wisdom couldn’t save him. He hung himself in 2008. What does his speech have to do with my quantum project? This, perhaps: Some sages say that to understand quantum mechanics is to understand reality. A recent book on quantum mechanics, What Is Real?, delves into debates about the measurement problem, spooky action, wave functions and other quantum razzmatazz. But how real is that stuff compared to what David Foster Wallace talks about? What does quantum mechanics, or physics generally, tell us about the horrors of modern life?
David Foster Wallace often wrapped a white bandana around his head, as if covering a wound.
Another thought. David Foster Wallace was a professional seeker, trying to find something of value to hold onto, to make life worth living. He possessed a powerful intellect and imagination, an ability to confront things head on, to see through the bullshit of others and of himself, to express how he felt and how he imagined others felt. His penetrating vision was his hope for salvation, but it was also what he needed saving from. It was his tragic flaw.
Why does the world delight one seeker while driving another to despair? It’s the same world, isn’t it? The Wigner’s-friend experiment seems relevant here. There is no correct, objective way to see this meaningless, meaning-drenched, brutal, delightful, heartbreaking world. David Foster Wallace is the friend trapped in a room with his despair. We stand outside the room, worried about him, afraid to open the door.
Are All Numbers Imaginary?
Jim Holt, after I whine about the kludginess of quantum mechanics, tells me that it only seems kludgy because I don’t see it clearly. He urges me to check out The Structure and Interpretation of Quantum Mechanics by philosopher R.I.G. Hughes. Hughes’s book is one long argument against the complaint that quantum mechanics is kludgy and ad hoc. Hughes is a graceful, intelligent writer, but reading him is frustrating, because his meaning usually eludes me. The poetry of John Ashbery evokes the same feeling in me, because I know my befuddlement is my fault, not his.
Every now and then I get a glimmer of understanding—for example when Hughes argues that Hilbert spaces are peculiarly suited to representing quantum things. Remember that Hilbert spaces aren’t spaces in the ordinary sense with width, length and depth, like your bedroom or the sky. They are habitats for vectors, which can describe anything from quarks to criminal justice in Cleveland.
Let’s say you represent the state of an electron with a vector, V, in a Hilbert space with three orthogonal axes, x, y and z. If you project V onto the x, y and z axes, you get the vectors Vx, Vy and Vz. “Pythagoras’ theorem tells us,” Hughes writes, that V² = |Vx|² + |Vy|² + |Vz|². Really? The Pythagorean theorem works in three dimensions? I never knew that.
Also, those vertical lines bracketing the Vs (which are not the same as the brackets around Dirac’s bras and kets) mean you’re taking the absolute value of each vector. Why? Shouldn’t squaring the vectors make them positive? No, because quantum vectors are often expressed as complex numbers, which contain imaginary numbers, based on the square root of -1. That’s why you need those vertical lines.
Anyway, these squared vector values, |Vx|², |Vy|² and |Vz|², represent the probabilities that the electron will be in a particular state when you measure it. The electron must be in some state, so all these fractional probabilities must add up to 1. 1 is also the value of V, which can be thought of as the hypotenuse of that three-dimensional analog of a triangle. If V = 1, then so does V². So |Vx|² + |Vy|² + |Vz|² = V² = 1. Getting the probabilities to equal one is called normalization.
Is the Pythagorean theorem, which is quite simple, really, the key to everything?
Everything is starting to make sense. All these seemingly arbitrary mathematical rules are based on the Pythagorean theorem plus probability theory. But “make sense” just means that the concepts are familiar; after a while, even the guy in the Chinese room starts recognizing strings of characters, and his recognition gives him a simulacrum of comprehension. And Hughes keeps undercutting himself; rather than trying to convince me that imaginary numbers are real, he mischievously suggests that real numbers are imaginary.
“If the inclusion of imaginary numbers is worrying,” he writes, “it is worth considering the sense in which a negative number, –6 say, is real—or, come to that, the sense in which 6 itself is real.” Hughes cites Bertrand Russell’s definition of mathematics as “the subject in which we never know what we are talking about, nor whether what we are saying is true.” Hughes’s irony throws me. Is his book one long joke? His grand finale is that quantum theory describes only “latencies,” which “can never be reduced to properties.” Really? That’s the big payoff? Latencies? [4]
The Wave Function of America
As if to mock my efforts to stay detached from the shitshow of America, an email list I’m on, which usually discusses wacky physics theories, has careened into wacky political theories. The list’s ringleader, whom I’ll call Snidely, has convinced others that he is a genius, who has discovered a way to communicate and even travel at faster-than-light speeds. Snidely is an avid Trump devotee and conspiracy theorist, as are others on his list, many of whom also have backgrounds in physics.
I keep asking to be removed from the list, but the nutty, nasty emails keep coming. Recently, Snidely and his minions have been feeding each other “evidence” that Chinese commies have orchestrated the Antifa and Black Lives Matter protests, and of course the Covid-19 pandemic, to harm Trump’s re-election chances. China hates Trump because he is a true patriot, unlike the cowardly commie-lover Biden. And so on.
These rants prove that training in physics does not make you rational. Not that I needed proof. If a conspiracy theory is a wacky explanation for the apparent chaos of existence, physicists espouse all sorts of conspiracy theories. The many-worlds hypothesis says universes with replicas of you and me are branching off our universe right now. And now. And now. Superdeterminism says Trump’s shenanigans have been foreordained--fated!--since the beginning of time. The simulation hypothesis says we’re living in a computer game invented by a superintelligent hacker in…
Oh, I don’t give a shit about the superintelligent hacker. In this scary era, the spectacle of highly trained scientists spouting hifalutin bullshit isn’t funny, it’s depressing.
Notes on R.I.G. Hughes and Pythagorean theorem in Quantum Notebook #2, July 24, 2020. I’m also trying to understand sailing in terms of vectors.
Craving fresh air, I stuff Hughes’s book into my backpack, put on a face mask and leave my 11th floor apartment. The elevator stops a few floors below mine, and a young man in a muscle shirt steps boldly on, wearing no mask. Our building requires masks in elevators. I hold my hand out like a traffic cop and say, You need a mask. He s-l-o-w-l-y takes a mask from his pocket and pulls it over his face, saying, Happy? I reply, No, you’re supposed to put it on before you get on the elevator.
Before he steps on the elevator, this guy is in a superposition of states. Latencies. But as soon as we interact, his wave function collapses into jerkitude. He’s probably a Trumper, who thinks that the pandemic is a hoax, a conspiracy to make Trump look bad. We glare at each other the rest of the way down. My blood is still boiling when I arrive at the park, a grassy pier that juts into the Hudson River. I find a shady spot between several chattering young families and assemble my collapsible chair. Soon I’m so immersed in Hughes that I forget about the jerk on the elevator and the conspiracy theorists writing me emails.
Except I can’t forget. I keep looking up from my book to glance at the Hudson, its surface crisscrossed by waves, and at the exuberant moms, dads and kids around me. It is the kind of sparkly day that makes it hard to imagine all the misery out there, all the anger and fear and despair. But I can’t banish my bad thoughts.
What if you could construct a wave function for this fucked-up real-imaginary country, the United States of America? What would it show? It would show us suspended in a superposition of many possible states, latencies, from okay to very, very bad. This fall, the Presidential election will serve as a test, a measurement, that collapses the wave function of America and reveals who we really are.
Notes
“Horganism” is not the only derogatory coinage based on my name. Two mathematicians incensed by my 1993 Scientific American article “The Death of Proof,” which argued that conventional proofs might become obsolete, named a geometric object after me, the Horgan minimal surface. A minimal surface is the smallest possible area bounded by a closed curve. The Horgan surface is actually a pseudo-surface, which has hidden gaps in it. The discoverers of the pseudo-surface meant to insult me by naming it after me, but I was honored. Plus, mathematicians have discovered Horgan surfaces that are genuine minimal surfaces, not pseudo-surfaces. I have been immortalized in the Platonic realm of forms and pseudo-forms!
I got help on the section following “Jargon alert!” from physicist/philosopher Jacob Barandes.
I wrote a column about the Chinese room experiment in early 2021 and got great responses. Here’s one, slightly abridged, from Winston Chang: I have a relevant real-life experience with the Chinese Room thought experiment that you might find interesting. I am an American born Chinese, ABC as some put it, who doesn’t really speak or comprehend Chinese. I have a few phrases and words I know, but I cannot carry or understand a conversation. Despite having spoken it as a young child and attempted to study it a year in college, I am pretty useless. In 2015 I spent a month in China on a fellowship. I typically had a translator with me wherever I went, but after work hours I took opportunities to wander around alone to better experience the country and people, unfiltered. Because I am ethnically Chinese, I had many encounters with people who assumed I was a native speaker. Most of these encounters were me nodding, flailing my hands, and trying to speak what few words I know. From a mental state they reminded me of going down stairs and trying to think about where to place my feet. However I had a few conversations where, for reasons unknown, my mental state was calm. In those conversations I could respond to the people in Chinese. In fact, sometimes it took a few minutes of conversing for those speaking to me to realize I wasn’t native. When these zen like states broke, the facade came tumbling down and they recognized me for the foreigner I was. Here is the tie back - I didn’t know what I was saying most of the time. The input and my own output was literally a foreign language to me. I comprehended neither.
In response to my persistent whining about the “kludginess” of quantum mechanics, Jim Holt sends me a lecture by quantum-computing expert Scott Aaronson, who says the “conceptual core” of quantum mechanics is a “generalization of probability theory to allow minus signs.” Aaronson acknowledges that the concept of negative probabilities sounds weird. “There's a reason you never hear the weather forecaster talk about a -20% chance of rain tomorrow--it really does make as little sense as it sounds.” But Aaronson notes that in quantum calculations, negative numbers ultimately generate only positive probabilities. Holt, when I ask him to clarify Aaronson’s remarks, emails me the following:
Mathematically, there are infinitely many schemes for probability. Of that infinity, however, only two of the schemes are compatible with a reality that evolves over time in a non-trivial way. One of these two schemes is classical probability--the probability of weather and casinos and presidential elections. The other is quantum probability--the probability of the microworld. Classical probability uses "real numbers," which are familiar to us all. Quantum probability uses "complex numbers," whose properties give rise to most of what seems weird to us about the quantum world. And it is the phenomenon of entanglement that ties these two probability schemes together: entanglement makes the quantum probabilities of the microworld unite to behave as the classical probabilities of the world of weather/casinos/ presidential elections. Seen in this way, nature is much less arbitrary than it might appear. There are only two viable schemes of probability. Nature avails itself of both; and, through entanglement, it ties them together in a neat package.
Table of Contents
INTRODUCTION
Old Man Gets More Befuddled
CHAPTER ONE
The Strange Theory of
You and Me
CHAPTER TWO
Laziness
CHAPTER THREE
The Minus First Law
CHAPTER FOUR
I Understand That
I Can’t Understand
CHAPTER FIVE
Competence Without Comprehension
CHAPTER SIX
Reality Check
CHAPTER SEVEN
The Investment Principle
CHAPTER EIGHT
Order Matters
CHAPTER NINE
The Two-Body Problem
CHAPTER TEN
Entropy
CHAPTER ELEVEN
The Mist
CHAPTER TWELVE
Thin Ice
CHAPTER THIRTEEN
Irony
EPILOGUE
Thanksgiving